方べきの定理の練習問題
本記事には,方べきの定理を使って解ける基本的な問題と解答例があります.
方べきの定理をしっかりと使いこなせるようになりましょう.
方べきの定理が分からない人は以下の記事をご参照ください.
問題1
以下の図の x の値を求めよ.
(1)
(2)
(3)
(4)
(5)

問題2
ある円に2本の弧 \mathrm{AB} と弧 \mathrm{CD} がある.この2本の弧が円の内側の点 \mathrm{E} で交わり,\mathrm{AB} = 18 ,\mathrm{AE} = 6 ,\mathrm{CE} = 8 であるとき,線分 \mathrm{DE} の長さを求めよ.
問題1の解答例
方べきの定理について簡単に復習しておきます.
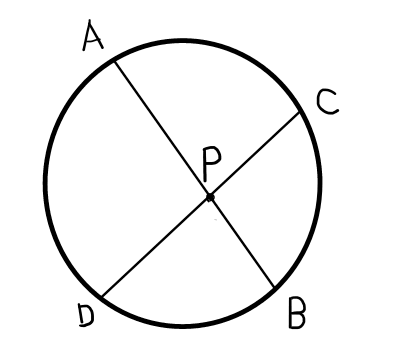
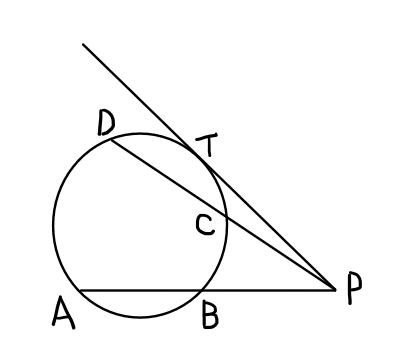
方べきの定理.
2本の弧 \mathrm{AB} と弧 \mathrm{CD} の交点を \mathrm{P} とするとき,以下の式が成り立つ.
\mathrm{AP} \cdot \mathrm{BP} = \mathrm{CP} \cdot \mathrm{DP}
また,上の図のように円の接線と弧 \mathrm{AB} の交点を \mathrm{P},接点を\mathrm{T} とするとき以下の式が成り立つ.
\mathrm{AP} \cdot \mathrm{BP} = \mathrm{PT}^2
それでは,解答例に移りたいと思います.
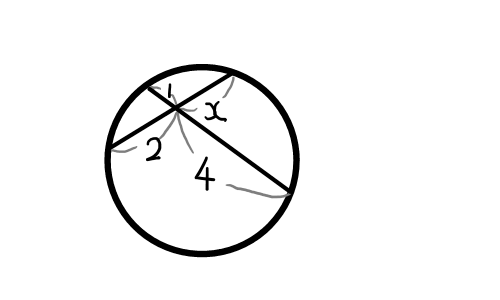
(1)

解答例.
方べきの定理より
\begin{aligned}
2 \cdot x &= 1 \cdot 4 \\
2x &= 4 \\
x &= 2
\end{aligned}
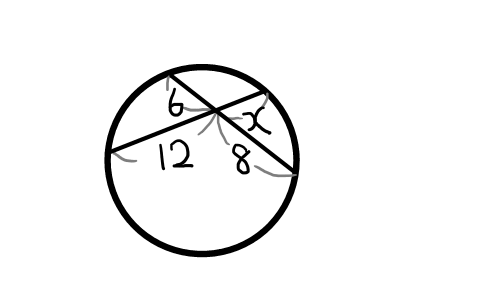
(2)

解答例.
方べきの定理より
\begin{aligned}
12 \cdot x &= 6 \cdot 8 \\
12 x &= 48 \\
x &= 4
\end{aligned}
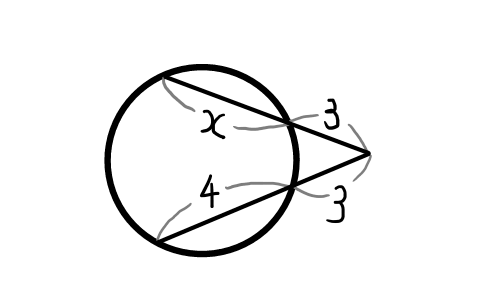
(3)

解答例.
方べきの定理より
\begin{aligned}
3 ( x + 3) &= 3 (3 + 4) \\
3 x + 9 &= 21 \\
3 x &= 12 \\
x &= 4
\end{aligned}
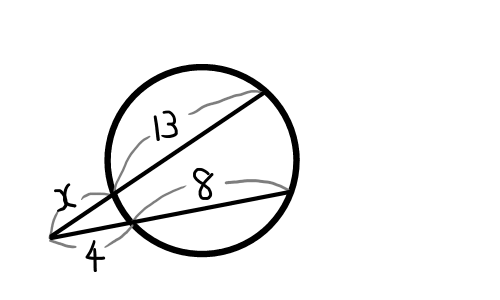
(4)

解答例.
方べきの定理より
\begin{aligned}
x ( x + 13) &= 4 ( 4 + 8 ) \\
x^2 +13x &= 48 \\
x^2 +13 x - 48 &= 0 \\
( x - 3 )( x + 16 ) &= 0
\end{aligned}
x > 0 より
x = 3
(5)

解答例.
方べきの定理より
\begin{aligned}
x^2 &= 9 (9 + 7) \\
x^2 &= 3^2 \cdot 4^2 \\
\end{aligned}
x > 0 より
x = 12
問題2の解答例
方べきの定理が使える図形の問題を,文章に変換しただけの問題です.
解答例.
\begin{aligned}
\mathrm{BE} &= \mathrm{AB} - \mathrm{AE} \\
&= 18 - 6 \\
&= 12
\end{aligned}
方べきの定理より
\begin{aligned}
\mathrm{CE} \cdot \mathrm{DE} &= \mathrm{AE} \cdot \mathrm{BE} \\
8 \cdot \mathrm{DE} &= 6 \cdot 12 \\
8 \mathrm{DE} &= 72 \\
\mathrm{DE} &= 9 \\
\end{aligned}

